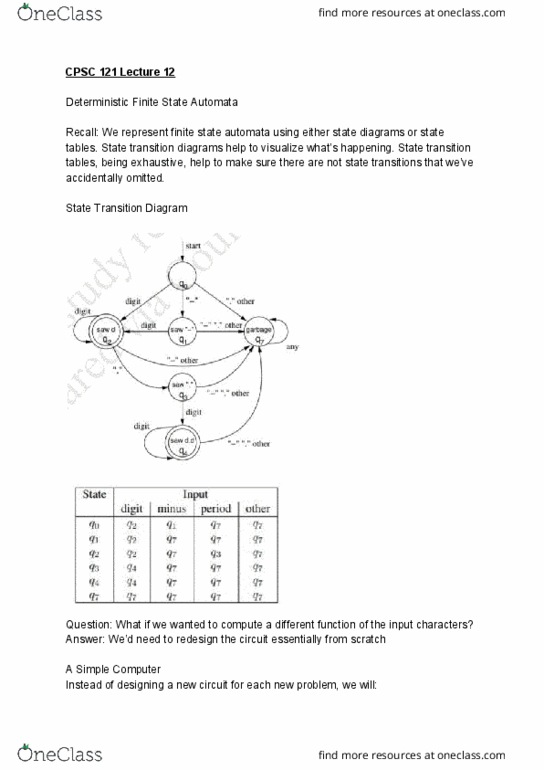
CPSC 121 Lecture Notes - Lecture 11: Universal Generalization, Rational Number, Universal Quantification
CPSC 121 verified notes
11/12View all
Document Summary
Prove that there exist irrational numbers x and y such that x^y is rational. Let r(x): x is a rational number (i. e, xeq) Most of the deductive arguments are examples of direct proofs. To prove this type of theorem, we must show that p(x) q(x) is always true. P(x) q(x) is false only if, for some x, p(x) is true and q(x) is false. Thus, we assume p(x) to be true and show q(x) must be true when p(x) is true. Aside: we don"t care if p(x) is false since p(x) q(x) then is true (vacuously) To summarize, the steps in a direct proof are: 1: assume that x is an arbitrary element of d for which p(x) is true, then, under this assumption, show that q(x) is true (using a valid argument with p(x), axioms, lemmas, and all other theorems as premises) We choose an x that is an arbitrary element of d and prove p(x) q(x).