CH ENGR 102A Lecture 4: Conservation-Laws
ChE 102A, Thermodynamics I, Winter 2017
Instructor Vasilios I. Manousiouthakis
Copyright © Vasilios I. Manousiouthakis, January 2012
Conceptual Derivation of Conservation Laws
Consider a Composite Thermodynamic System
CSys
with mass
m
, that is a large
collection of simple thermodynamic systems
Sys
of infinitesimal size and mass
dm
. Consider
also that
CSys
is moving, occupying a different control volume
t
CV
at
t
than the control
volume
tt
CV
it occupies at an infinitesimal time
t
later, i.e. at
tt
. The control surfaces of
t
CV
,
tt
CV
are designated as
t
CS
,
tt
CS
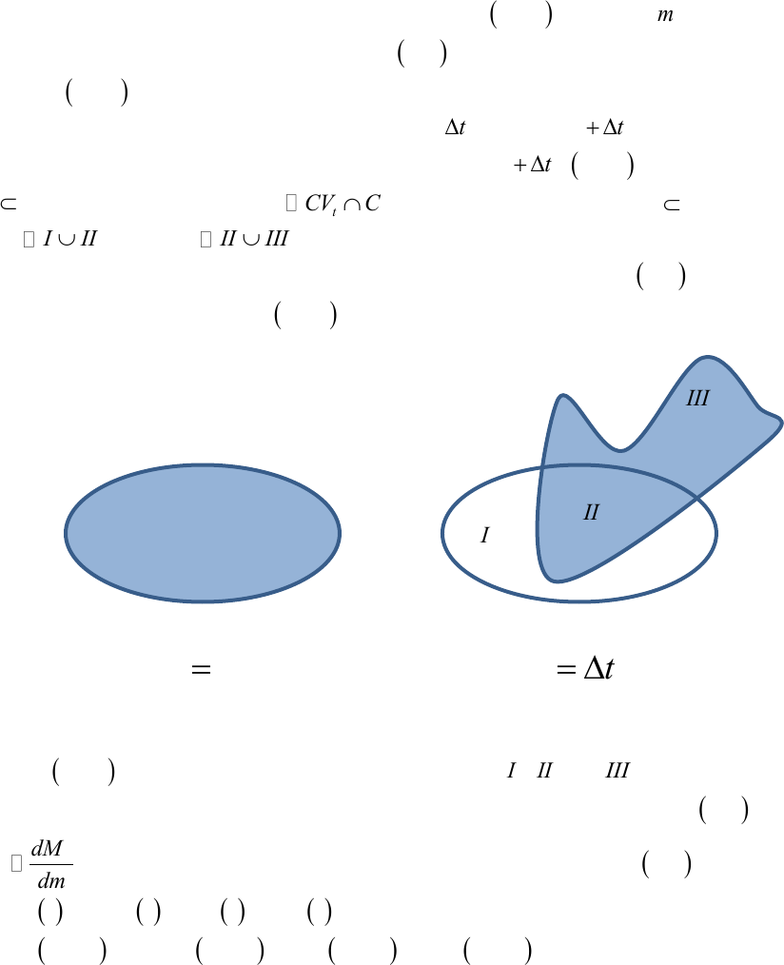
respectively. At
tt
,
CSys
has exited region
t
I CV
, still occupies region
t t t
II CV CV
and has entered region
tt
III CV
, where
t
CV I II
and
tt
CV II III
.
This allows the thermodynamic properties of each infinitesimal
Sys
to be associated
with each point occupied by the
CSys
.
Let
t
CSys
M
,
t
t
CV
M
,
tt
t
CV
M
,
t
I
M
,
t
II
M
, and
t
III
M
denote an arbitrary extensive variable of the
system
CSys
and of the mass in the regions
t
CV
,
tt
CV
,
I
,
II
, and
III
respectively. Let also
t
dM
denote the corresponding extensive variable in each infinitesimally sized
Sys
, and
t
dM
Mdm
denote the corresponding specific intensive variable for each
Sys
. Then,
t
t t t t
CSys CV I II
M t M t M t M t
, and
tt
t t t t
CSys CV II III
M t t M t t M t t M t t
From the definition of a derivative in calculus, we can express the change in
t
CSys
M
:
0t
tt
CSys
CSys
III
II
I

0
lim
t t t
CSys CSys CSys
t
dM t M t t M t
dt t
0
lim
t t t t
t
II III I II
CSys
t
M t t M t t M t M t
dM t
dt t
0
lim
t t t t t t
t
II III I II I I
CSys
t
M t t M t t M t M t M t t M t t
dM t
dt t
0
lim
t t t t t t
t
I II I II III I
CSys
t
M t t M t t M t M t M t t M t t
dM t
dt t
0
lim tt
t t t t
t
CV CV III I
CSys
t
M t t M t M t t M t t
dM t
dt t
0 0 0
lim lim lim
tt
tt
ttt
CV CV
CSys III I
t t t
M t t M t
dM t M t t M t t
dt t t t
00
lim lim
t
t
ttt
CV
CSys III I
tt
Mt
dM t M t t M t t
dt t t t
Consider now the intensive variable
called mass density and defined as
t
dm
dV
. Then
the intensive variable corresponding to the extensive variable of volume
t
V
is
1t
dV
dm
. Since
each
Sys
is infinitesimal in size,
can be considered as the image of the density function
3
: , , , , , , kg
t x y z t x y z m
. It then holds:
t
t
CSys CV
m t dm dV
t
t
t t t t
CSys CV
CSys CSys CV
M t M t dM Mdm M dV
, which in turn implies
t
t
t
t
CV
CV
M dV
Mt
tt
. Similarly
t t t
III
III III III
M t t dM Mdm M dV
t t t
I
I I I
M t t dM Mdm M dV
However, in the regions
I
,
III
and as
0t
it holds:
tt
dV v t ndA
,
tt
dV v t ndA
respectively. Then, the earlier derived equation for
t
CSys
dM t
dt
becomes:
0
00
lim lim
t
t
ttt
t
CV
CSys III I
tt
Mt
dM t M t t M t t
dt t t t
00
lim lim
tIII I
ttt
t
CV CS CS
CSys
tt
M dV M v t ndA M v t ndA
dM t
dt t t t
t
III I
t
t
CV
CSys tt
CS CS
M dV
dM t M v ndA M v ndA
dt t
tt
t
CSys tt
CV CS
dM t M dV M v ndA
dt t
The above equation is often referred to as Reynolds’ Transport Theorem (RTT).
From here, the derivation can be continued for two separate cases: first for a composite system
whose constituents are differential sized simple subsystems, and second for a simple system. The
first yields the conservation laws for transport phenomena, and the second yields the
conservation laws for thermodynamics.
a. Transport Phenomena
By invoking Gauss’ Divergence Theorem, the surface integral in the right hand side of
the above equation for
t
CSys
dM t
dt
can be expressed as a volume integral involving the dot
product,
, of the surface integral’s integrand with the
operator, i.e.
tt
tt
CS CV
M v ndA M v dV
.
In addition, considering the control volume
t
CV
fixed, Leibnitz’s theorem suggests that the
partial derivative and volume integration operators can be exchanged, i.e.. It then holds:
tt
t
CSys tt
CV CV
dM t M dV M v dV
dt t
t
t
CSys t
CV
dM t M M v dV
dt t
Allowing the control volume
t
CV
to become infinitesimally small yields
t
tt
CV
V dV
and
makes the integrand spatially uniform. Thus it can be taken out of the integral leading to:
Document Summary
Csys with mass m , that is a large collection of simple thermodynamic systems also that volume. Cv it occupies at an infinitesimal time. Csys is moving, occupying a different control volume t later, i. e. at t t , . Cv are designated as tcs , t t t t t t. Sys of infinitesimal size and mass dm . Consider tcv at t than the control t . Iii cv and has entered region t. This allows the thermodynamic properties of each infinitesimal . Ii t t t with each point occupied by the . Csysm , system tdm denote the corresponding extensive variable in each infinitesimally sized . Csys and of the mass in the regions. Im , t t t t t t t t. Cv , i , ii , and iii respectively. Iiim denote an arbitrary extensive variable of the.

