Applied Mathematics 2270A/B Lecture Notes - Lecture 6: Bernoulli'S Principle
28 Sep 2018
School
Department
Professor
Recall:
Seperable
1.
1st order linear
2.
Exact
3.
You know how to solve DEs that are
Solving DEs by Substitution (Bernoulli Equation & Homogeneous DE)
If the equation is too difficult, we can try to change variables to make it easier!
"A method to change the problem into one we already know how to solve"
Bernoulli's Equation
Equations of the form
dy + P(x) y = Q(x) y-n
dx
You aren't supposed to
know what the substitution
is immediately. It takes time
and trial/error and
frusteration. It just takes
time
2.5 Lecture
September 24, 2018
9:35 AM
Lecture Notes Page 1
n= 0 or n = 1, 2, 3 ... -> First order linear
For n ≠0 & n ≠1, we can use V = y1-n
Bernoulli figured out that there is a natural substitution you can make for n = 1, 2, 3, ...
Derivation
dv = (1-n)y-n dy
dx dx
dy = yn dv
dx (1-n) dx
The Bernoulli Equation
dv + (1-n) P(x) V= (1-n) Q(x)
dx
This is Ordinary, 1st order linear
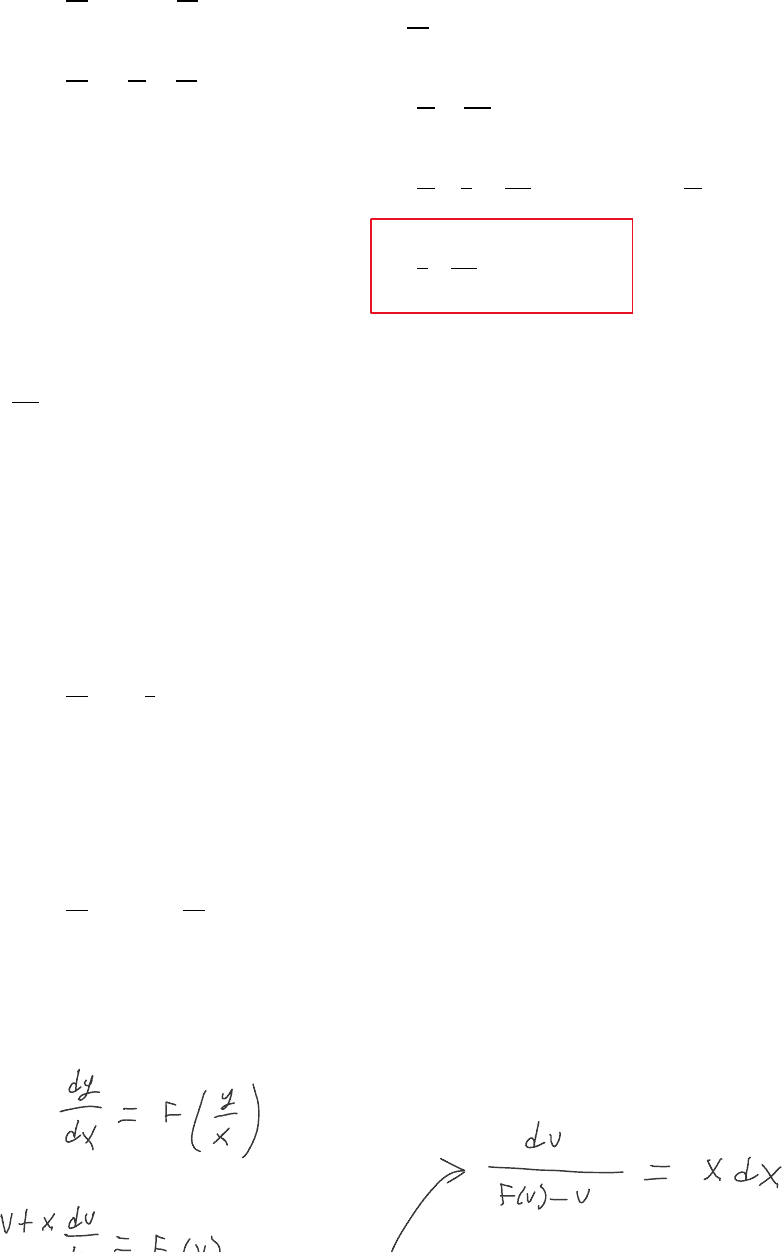
Homogeneous ODE (1st order)
dy = F( y )
dx x
Def: A homogeneous, 1st order ODE is one which may be written as
V = y/x
y = vx
dy = v + x dv
dx dx
In these cases the "proper" (useful) substitution
Then substitute
Sub into ->
dy + P(x) y = Q(x) y-n
dx
yn dv + P(x) y = Q(x) y-n
(1-n) dx
yn 1 dv + P(x) y1-n = Q(x) yn
yn (1-n) dx yn
1 dv + P(x) V= Q(x)
1-n dx
Lecture Notes Page 2
Document Summary
You know how to solve des that are. Solving des by substitution (bernoulli equation & homogeneous de) If the equation is too difficult, we can try to change variables to make it easier! "a method to change the problem into one we already know how to solve" You aren"t supposed to know what the substitution is immediately. Equations of the form dy + p(x) y = q(x) y-n dx. Lecture notes page 1 n= 0 or n = 1, 2, 3 -> first order linear. For n 0 & n 1, we can use v = y1-n. Bernoulli figured out that there is a natural substitution you can make for n = 1, 2, 3, Derivation dv = (1-n)y-n dy dx dx dy = yn dx (1-n) dx dv. Sub into -> dy + p(x) y = q(x) y-n dx dv + p(x) y = q(x) y-n yn (1-n) dx.
