MATH-M 303 Lecture Notes - Lecture 11: Gaussian Elimination, If And Only If, Linear Independence

• School: IU
• Department: Mathematics
• Course: MATH-M 303
• Professor: Kidwell Keenan

Document Summary

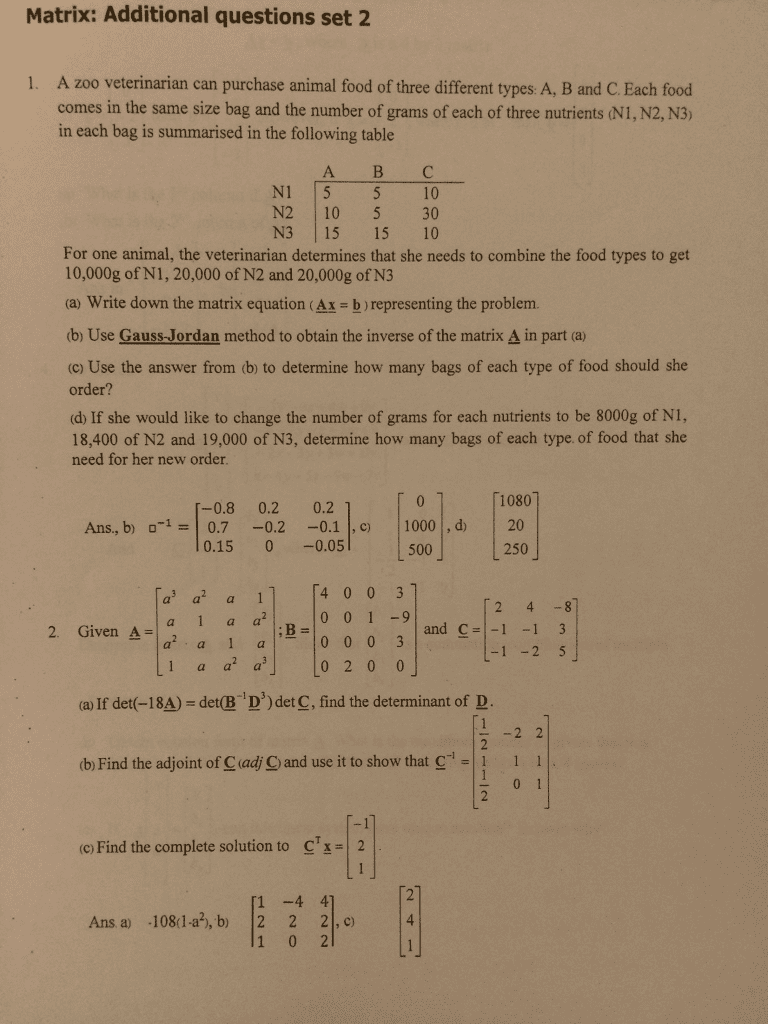
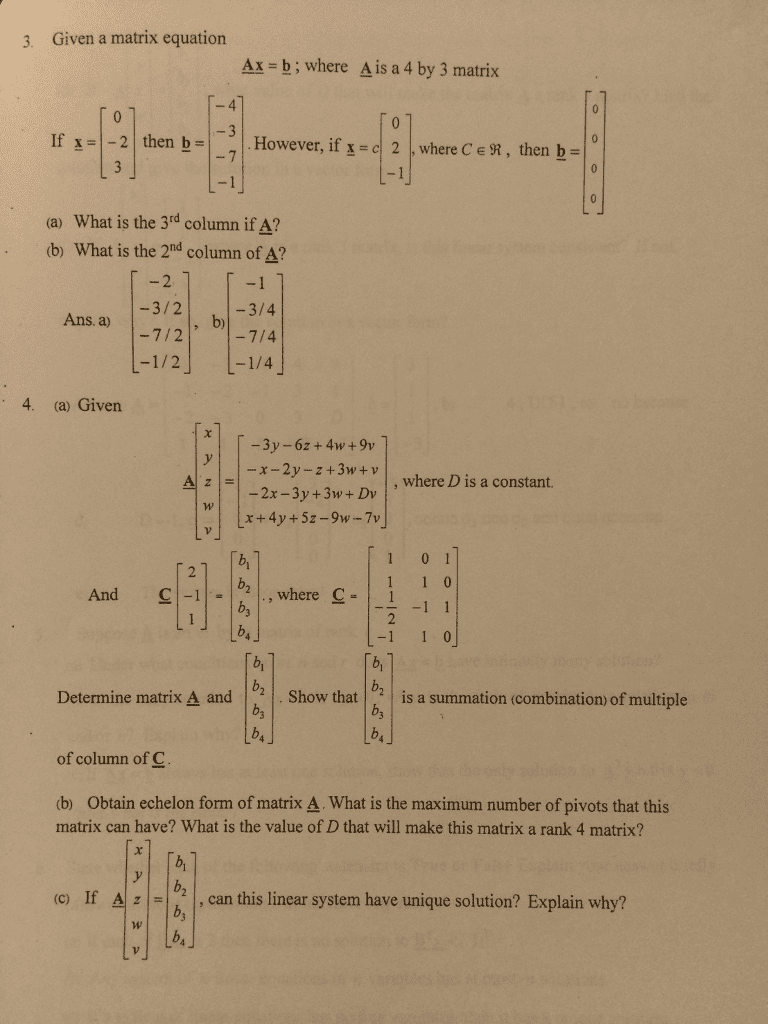
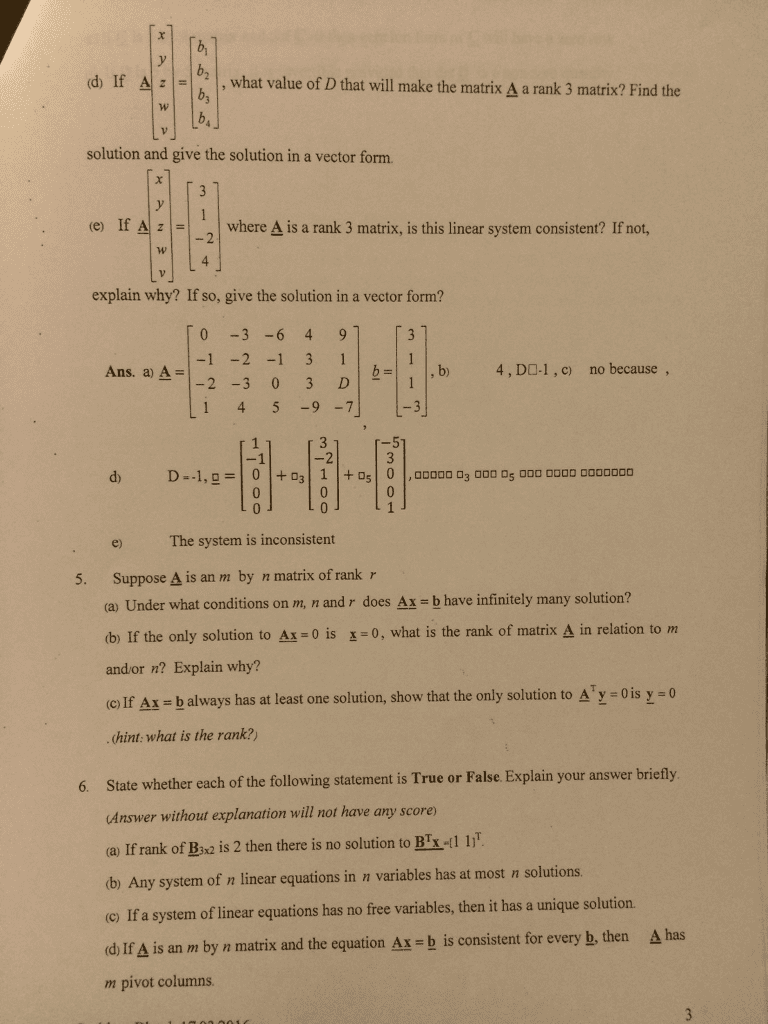
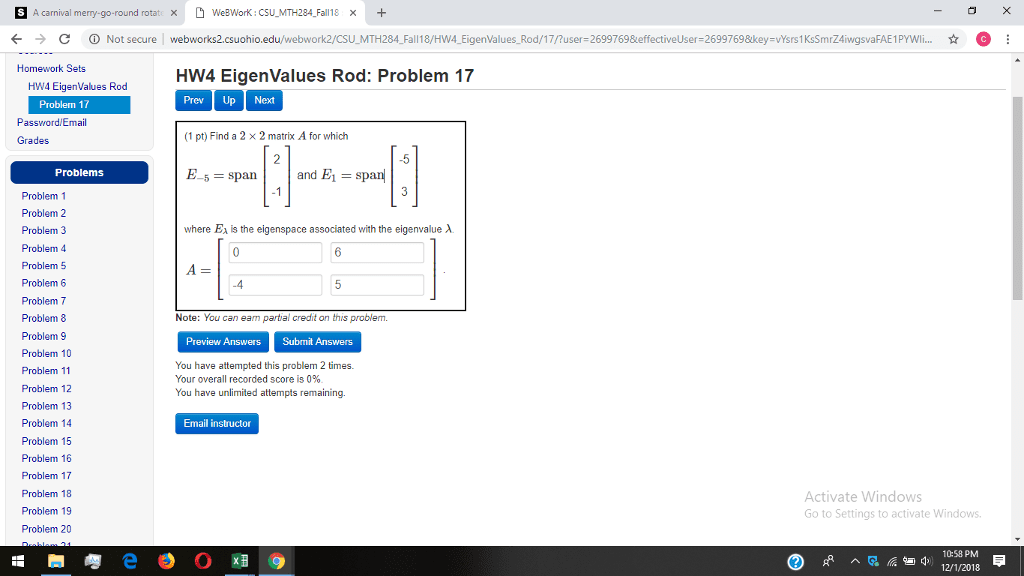
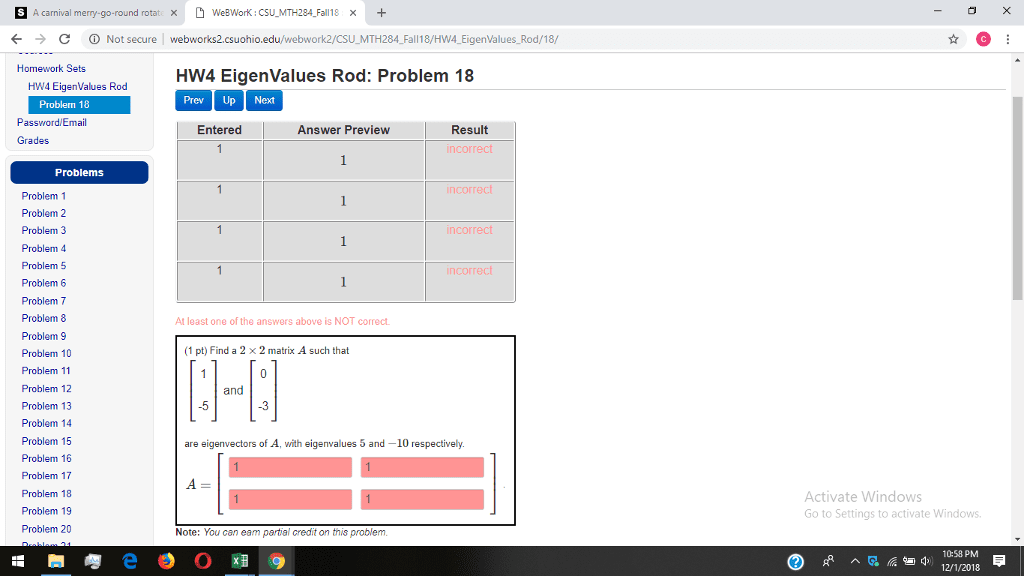
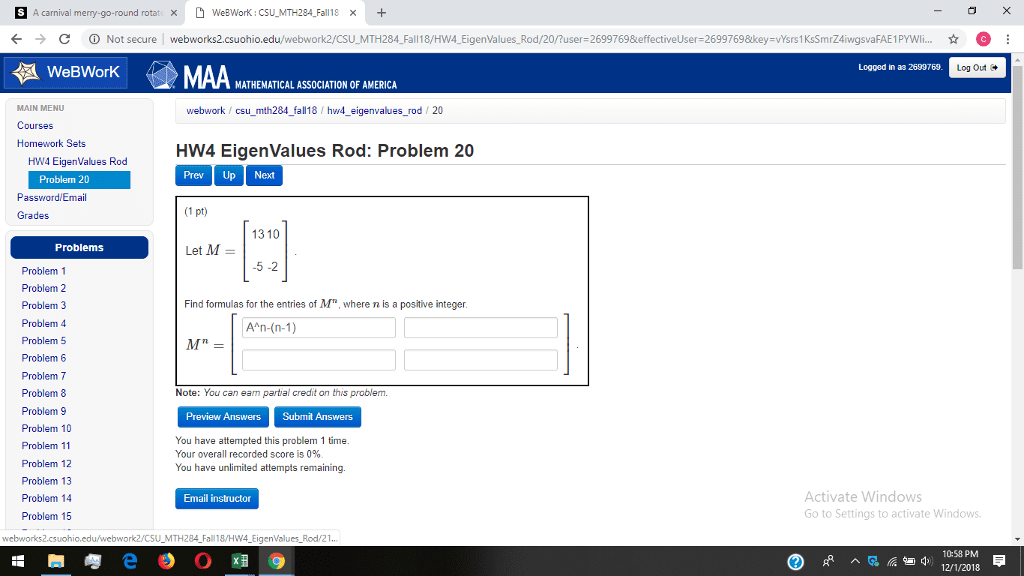
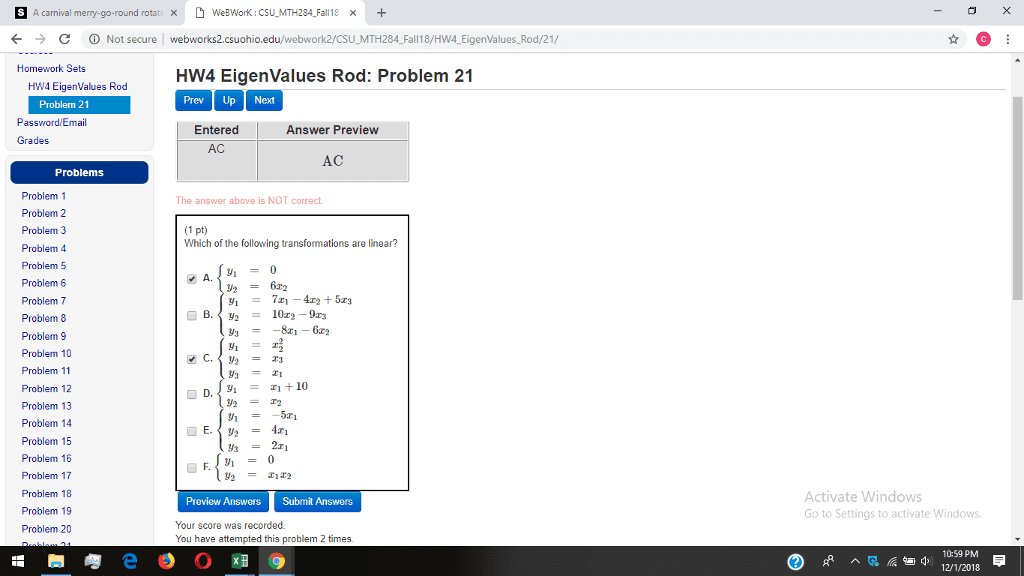
Unlike real (nonzero) numbers, not every matrix has multiplicative inverse. (cid:1866) (cid:1866) matrix (cid:1827) invertible/nonsingular if there exists another (cid:1866) (cid:1866) matrix (cid:1829) such that (cid:1827)(cid:1829)==(cid:1829)(cid:1827: (cid:1829) unique and defined as inverse of (cid:1827), denoted (cid:1827) (cid:2869, to check that (cid:1829)=(cid:1827) (cid:2869), only have to check one equality above. Invertible matrices tell us about invertibility of linear maps (whether is 1-1 and/or onto) For (cid:1827)=[(cid:884) (cid:887) (cid:884)], show that (cid:1829)=(cid:1827) (cid:2869). (cid:885) (cid:885) (cid:889)][ (cid:889) (cid:887: (cid:1827)(cid:1829)=[(cid:884) (cid:887) (cid:884)] (cid:885) Theorem 4- let (cid:1827)=[(cid:1853) (cid:1854) (cid:1855) (cid:1856)]; if dt(cid:1827)=(cid:1853)(cid:1856) (cid:1854)(cid:1855) (cid:882), then (cid:1827) invertible and (cid:1827) (cid:2869)= (cid:2869)(cid:2914)(cid:2915)t[(cid:1856) (cid:1854) (cid:1853)] Show that (cid:1827)=[(cid:885) (cid:886)(cid:887) (cid:888)] is invertible and find (cid:1827) (cid:2869). Theorem 5- if (cid:1827) is invertible (cid:1866) (cid:1866) matrix, then (cid:1827)=(cid:2184) always has unique solution =(cid:1827) (cid:2869)(cid:1828) for each (cid:2184) . Theorem 6- properties of (cid:1866) (cid:1866) matrix inverses. If (cid:1827) invertible, then (cid:1827) (cid:2869) also invertible and (cid:4666)(cid:1827) (cid:2869)(cid:4667) (cid:2869)=(cid:1827)